Dynamics III – 4.3 Resistance

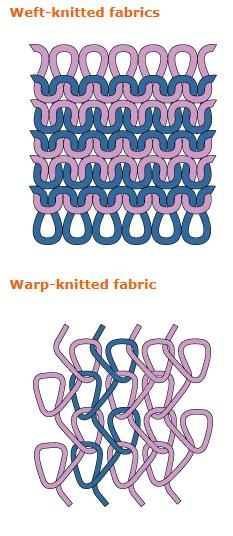
The Cloth Room Resistances for Fold, Shear and Stretch are determined by the fibers used, and the weave in which the fibers are combined.

The weaves – some of them shown in the next page figure – are hard to make calculations for. Fabric parameters themselves are published and available on the net, but only for the heavy duty / high performance ones. Look for fabrics for game sailing or surfing or parachutes, and you’ll find plenty. They even use different weaves for the different sails on the same ship. Look for fabrics for normal clothing, and you’ll find none. Google for “stretching jeans” and you’ll get lots of tips how to make you pants fit better. Google “folding cotton” and you’ll get a course in fancy towel folding.

But that’s for single fiber, and usually fibers are spun into yarn, with thicker threads, thicker cloth and higher tex values. For various silk fabrics, values can be found like:

• Gauze     12 to 20 gr/m²
• Organza     15 to 25 gr/m²
• Habutai      20 to 70 gr/m²
• Chiffon      25 to 35 or 50 to 70 gr/m² (double thickness)
• Charmeuze     25 to 125 gr/m²
• Crepe de Chine     50 to 70 gr/m²
• Raw Silk    150 to 175 gr/m²

Note 1: thickness affects appearance: 10 gr/m² is semi transparent, 25 gr/m² is translucent, 100 gr/m² is opaque.
Note 2: To put values in perspective: Poser default cloth density reads 0,005 gr/cm² = 50 gr/m².

Now we can start to pull the fiber. The result of that depends on the force per fiber-cross section, in Newton per m², or more practical: N / mm². But since fibers can come in various thicknesses, N/tex is the preferred material constant. And the amount of N/tex times the Specific Weight (in gr/cm³) of the material results in kN/mm²:

5 N/tex * 1.2 gr/cm³ = 5 N / ( 1 gr / 1,000,000 mm) * 1.2 gr/ 1000mm³ = 5 * 1,000,000 / 1000 * 1.2 N/mm² = 7 kN/mm².

At low forces, the elasticity or modulus of the material is the ratio between the stretch in % and the force in N/mm2. At high forces, a specific amount of N/mm2 will make the fibers snap. By doubling the thickness of the fiber (thread, yarn, cable) one can quadruple the strength of it.

Since measurements usually have a more scientific / engineering background instead of an industrial one, fiber strength is expressed in MPa (MegaPascal, 1 Pa = 1N/m²) or psi (pound/square inch).
1 N/mm² = 1 MPa = 146.25 psi; 1 kpsi= 6.84 MPa.

Values for polyester (www.ides.com):

• Specific weight (aka “gravity”): 1.24 to 1.48 g/cm³.
• Tensile Modulus (stiffness): about 300,000 to 400,000 psi or: 3000 to 4000 psi per % elongation
  that’s 3000 to 4000/146.25 = 20 to 30 N/mm² per % (or say 25/1000/1.25 = 0.02 N/tex)
  The breaking strength is 5000 to 9000 psi or about 35 to 60 N/mm² which is about twice as much, so polyester is not going to stretch very much.

Steel has about a similar stiffness (20 N/mm per % elongation) and due to its high specific weight (8 g/cm³) a low 0.0025 N/tex value: you’ll get less stretch resistance per pound of material. But it stretches nicely till say 200 MPa and breaks at 400 MPa. So steel can handle far larger forces than polyester, and stretches up to 10%.
Lead for instance is much more deformable, with 1.6 N/mm² per % elongation.

Teflon / PTFE: 2.2 gr/cm³, breaking strength 28 N/mm²; it’s a heavy, brittle material but it has an extremely low friction: 0.1 which makes it fine for surface coatings.

Another example: Kevlar (as in bullet proof vests): The modulus is about 60N/mm per % strain (twice as strong as polyester), but breaking at 2N/tex, and with a SW = 1.45 g/cm³ we get 2900 N/mm². That’s why it’s in bullet proof vests: the projectile has to break the fibers and that slows it down considerably, it’s say 10 times stronger than steel. Human skin breaks at say 20 N/mm² so that’s why we need the protection.

High performance cabling for shipping, like Astra: 0.97 gr/cm³ (so it floats on water), and with 0.15 – 0.20 N/tex it can handle about 150 N/mm² while stretching only 1%. And is can stretch a lot (30% or so) while not breaking. This is the stuff that keeps ships to the quay, especially when using over 30 mm thick cables.

Generally speaking, the stiffness for high performance cabling is about 100 N/mm² per % elongation. For most materials that we make to cover and protect our body, the stiffness is about 10. For natural clothing materials, (wool, cotton) it’s about 1. I needed tens of pages on the net to gather and combine information like the above. And I still have nothing reasonable for fabric itself, I’ll have to construct that.

1 meter of cloth required about 1000/(1.5 d) threads in one direction, and about the same in a perpendicular direction, using thread diameter d in mm. When a single thread has a stiffness S (eg 25 N/mm² per % elongation) then 1 m of cloth has a stiffness of (1000 S)/(1.5 d)*(3 d²/4) = S*d*500 (pi rounded to 3 as the 1.5 thread distance is a quesstimate anyway).

I also stated above that 1 m2 of fabric has a weight of 4T/3d, in gr/m², diameter d in mm, T in tex about equal to 1000 d², so the weight in gr/m² equals about 4.000 d/3 (d in mm). In Poser, I concluded earlier that the ratio Stretch Resistance to Cloth Density is typical for the material, an in this case it reads (S*d*500) / (4000 d/3) = 1.5 S / 4. In nature, that is say 10 and in Poser (default values) that is 50 / 0.005 = 10,000. Let’s check units again.

Assume d=1mm, then 1 m cloth takes 1000/1.5 = 667 threads, each having a 3/4 mm² cross section. Stiffness S = 25 N/mm² per % elongation then turns into 667 * 3/4 * 25 = 12,506 (N/mm² per %). The amount of fiber is 2* 667 m * 3/4 mm² = 1000 cm³, and hence weights 1340 gr (/m²). Which sounds okay, considering a 1mm thick thread resulting in a say 2mm thick cloth. The stiffness / density ratio is 12,506/1340 = 9.33 about 10.

In Poser, cloth density is not measured in gr/m² but in gr/cm². This makes a factor 10,000. Second, the default stretch resistance is known to produce far too elastic cloth in the simulations, 500 might do far more realistic and in line with the real values we’re using here (although it might elongate the sim itself). So, in Poser: 500 / (0.005 * 10,000) = 10 again.

In other words, the Poser Stretch Resistance is: (1.5/4 * 10,000 =) 3750 * S * D for stiffness S (in N/mm per %) as found in the literature, and cloth density D (in gr/cm² as set in Poser). Realistic densities range from 0.025 to 0.075 despite the 0.005 default representing silk. Realistic stiffness values are:

• Rubber        0.3 – 1
• Natural fabric    1 – 3 (wool, cotton)
• Enhanced    3 – 10 (cotton / polyester etc)
• Artificial    10 – 30 (nylon, …)

A reasonable cloth value is: 4 (enhanced cotton) * 0.03 (thin shirt) * 3750 = 450. And when that turns out to be too high for a proper sim, reduce both Resistance and Density in sync.

Since shearing is meaningful for cloth only, and not for individual fibers, and has less industrial implications and applications compared to stretch, there is far less research done and there are far less values available. So my suggestion is to use values below the Stretch Resistance. Just a bit, except for those special cases that hardly stretch but shear a lot, like chain mail vests. Then Shear Resistance really is smaller than the Stretch one.

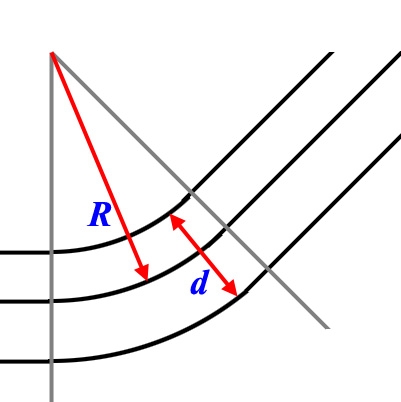
Folding has a similar story. The industry wants to know how many times a fiber can be folded before it wears out and breaks. Resistance to folding is futile. Actually, when folding a fiber with thickness d (diameter), it experiences a local stretching of 100 * d/R. 100 for making the result into %, and R is the folding radius, much larger than d.

As can be seen in the figure, the core of the fiber makes a turn forcing the outer part to relatively stretch d/2R and the inner part to shrink d/2R as well.

A sharp fold means a small R and much local stretching in the fibers. And we discussed stretching. Thicker cloth means thicker threads so d/R goes up for the same sharpness of the folds: it takes more effort to make them. Which material is a better folder? Hard to say, a better question is: which material folds sharper for the same fiber thickness and the same force applied?

Rubber seems a bad folder as it’s hard to make the folds really sharp, but what if it’s equally thick as a cotton shirt? Personally, I tend to follow the stiffness values as presented above. Rubbers fold better than natural fabrics over enhanced fabrics over artificial materials, at the same cloth (mass) density.

Since folding only affects a portion of the cloth instead of the whole cloth, using a Folding Resistance which is a tenfold smaller than the Stretch Resistance (as demonstrated in the defaults) might be a good idea.